182
My grandson brought me this example and asked if I could solve it, and I had to spend a lot of time trying to get old.
What do you remember from school? addition? Today I propose to refresh the memory of the main rules that relate to this topic. And, of course, we will do this by solving interesting mathematical examples. It's time to stretch the gyrus and unload the head!

Let us remember together how the addition of negative numbers works and what pitfalls there are in this topic. First, it is based on the mathematical rules and properties of arithmetic. Secondly, to add and subtract negative numbers correctly, you need to understand the signs of numbers.
Positive numbers are numbers greater than zero (e.g. 1, 2, 3, etc.). Negative numbers are numbers less than zero, they are denoted with a minus before the number (for example, -1, -2, -3). Zero (0) is neither positive nor negative.
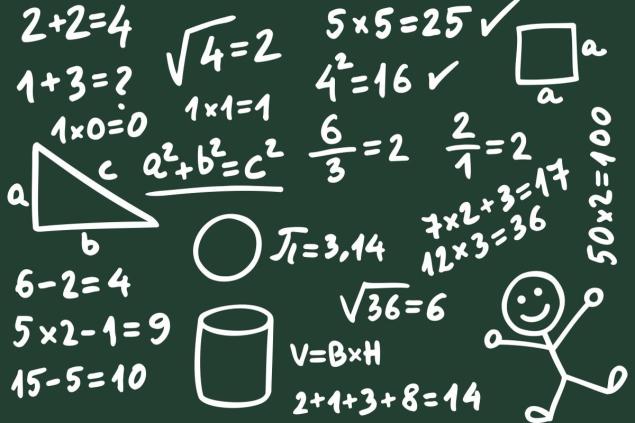
Adding two negative numbers works like this. When adding negative numbers, the result will also be a negative number. For example, (-3) + (-4) = -7. Adding positive and negative numbers is a bit more complicated. In this case, we subtract the absolute value of the negative number from the absolute value of the positive number and assign the result the corresponding sign. For example, 5 + (-2) = 5 - 2 = 3.
Adding a negative number and zero has its own rules. The result is a negative number, because zero will not change anything. For example, (-3) + 0 = -3.

Remember that when adding negative numbers, the result will always be either a negative number or a zero (if one of the terms is zero). For more complex expressions with negative numbers, use the rules of priority of operations (brackets, multiplication, division) and perform operations in turn, starting with the highest priority.
We remind you of how to add negative numbers. But what if one of these numbers first needs to be squared? Try to set an example that you see in front of you!

At first glance, the example seems simple. But the problem is that the people who solve it are divided into two camps. Some say the answer is 0, others say the solution is 200. Whose side is the truth on? Let's figure it out!
Here, as always, the presence of brackets is very important. Namely -10 in brackets. At this point, many come across, because with brackets and without them, the answer is completely different. The mathematical example is presented as follows: -102 + 100.

This means that you need to square the number 10, not -10. It's very easy to do that. Just multiply 10 and 10. The result is 100. However, the same minus has not gone anywhere. It comes out -100 + 100 = 0. That's the solution!
So why do so many people make 200? It's simple. They mistakenly square the number -10. Indeed, -10 * -10 = 100. Then 100 + 100 = 200. But to square the number -10, the example itself must look like this: (-10)2 + 100. We see in the picture a completely different condition.
Share in the comments what happened to you and do you understand the algorithm for solving this example?
Addition of negative numbers: another example Let's consolidate the material passed and solve a couple of similar examples.

-52 is -25. (-4)2 is 16, and (-3)2 is 9. It turns out -25 + 16 + 9 = 0.
That's all the challenges for today! Tell me, how do you train your brain in the hustle and bustle of everyday routine?

Let us remember together how the addition of negative numbers works and what pitfalls there are in this topic. First, it is based on the mathematical rules and properties of arithmetic. Secondly, to add and subtract negative numbers correctly, you need to understand the signs of numbers.
Positive numbers are numbers greater than zero (e.g. 1, 2, 3, etc.). Negative numbers are numbers less than zero, they are denoted with a minus before the number (for example, -1, -2, -3). Zero (0) is neither positive nor negative.

Adding two negative numbers works like this. When adding negative numbers, the result will also be a negative number. For example, (-3) + (-4) = -7. Adding positive and negative numbers is a bit more complicated. In this case, we subtract the absolute value of the negative number from the absolute value of the positive number and assign the result the corresponding sign. For example, 5 + (-2) = 5 - 2 = 3.
Adding a negative number and zero has its own rules. The result is a negative number, because zero will not change anything. For example, (-3) + 0 = -3.

Remember that when adding negative numbers, the result will always be either a negative number or a zero (if one of the terms is zero). For more complex expressions with negative numbers, use the rules of priority of operations (brackets, multiplication, division) and perform operations in turn, starting with the highest priority.
We remind you of how to add negative numbers. But what if one of these numbers first needs to be squared? Try to set an example that you see in front of you!

At first glance, the example seems simple. But the problem is that the people who solve it are divided into two camps. Some say the answer is 0, others say the solution is 200. Whose side is the truth on? Let's figure it out!
Here, as always, the presence of brackets is very important. Namely -10 in brackets. At this point, many come across, because with brackets and without them, the answer is completely different. The mathematical example is presented as follows: -102 + 100.

This means that you need to square the number 10, not -10. It's very easy to do that. Just multiply 10 and 10. The result is 100. However, the same minus has not gone anywhere. It comes out -100 + 100 = 0. That's the solution!
So why do so many people make 200? It's simple. They mistakenly square the number -10. Indeed, -10 * -10 = 100. Then 100 + 100 = 200. But to square the number -10, the example itself must look like this: (-10)2 + 100. We see in the picture a completely different condition.
Share in the comments what happened to you and do you understand the algorithm for solving this example?
Addition of negative numbers: another example Let's consolidate the material passed and solve a couple of similar examples.
- (-3)2 + (-2)2 =?

The algorithm is simple. First we will know how much (-3)2 will be. This (-3) * (-3) = 9 Then square the second number (-2)2. So (-2) * (-2) = 4 And then add these numbers and get 9 + 4 = 13. - -52 + (-4)2 + (-3)2 =?

-52 is -25. (-4)2 is 16, and (-3)2 is 9. It turns out -25 + 16 + 9 = 0.
That's all the challenges for today! Tell me, how do you train your brain in the hustle and bustle of everyday routine?
Before the guests arrive, I send a glass of this product to the toilet, the pollution disappears, the room fills with a pleasant aroma.
Take a look at this ripe corn, pick one and find out what the last month of summer has in store for you.