348
The efficiency of the motor increased due to a new method of optimization of the form
In the world of rapidly developing technologies, successful and cost-effective design of automotive and industrial components is crucial. The optimization of the geometry of individual parts of complex machinery increases the productivity and efficiency of the entire device as a whole.
To achieve this goal, automotive industry and aircraft industry often rely on filtering – a method that uses simulation to create the most simple and effective used in production designs. "Smoother rotation of the rotor can increase the energy efficiency of the engine and, at the same time, to reduce undesirable side effects, such as noise and vibration," says the mathematician Ulrich Langer (Ulrich Langer).
Professor Kepler University Linz Ulrich Langer, in collaboration with scientists, colleagues from Germany and Austria are the co-authors of scientific work published in the journal SIAM Journal on Scientific Computing, which describes the possibility of improving the performance of electric motors with optimized forms. "The optimal geometry of the motor, which previously was not possible even to imagine, you can now determine," says Langer.

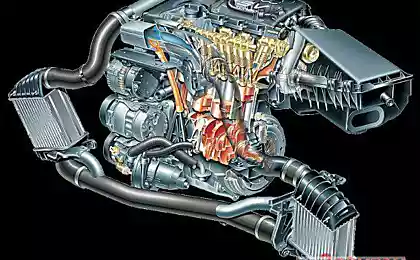
The optimization problem of the form, as a rule, are solved by minimizing a cost function – a mathematical formula that predicts the loss in accordance with the process. Lehner and his colleagues have used this method for interior permanent magnet (IPM) brushless motor, versions of which are sometimes used in washing machines, cooling fans, computers, etc., the Rotor includes iron core and permanent magnets. And since the geometry of all components of the rotor can be changed, the authors highlighted a subregion in the iron core of the rotor, the form of which may be subject to optimization. The result of the design, which allows to get more smooth and efficient rotation.
Scientists proposed an optimization procedure derived from the methods of Lagrange for the approximation of nonlinear problems, and demonstrate effective and accurate means of calculating the shape derivative from the cost function. This simple and comprehensive method allows to solve nonlinear differential equation with partial derivatives (PDE) and total cost functions. Ultimately, their optimization procedure is able to show a 27 percent decrease on the cost function IPM brushless motors, as demonstrated in a specific example of scientific work.
Now, when they effectively used the derivative of the shape optimization, the researchers hope to improve their method with further experiments. "Currently, we are working to create so-called "topological derivative" to solve such nonlinear optimization problems, says Peter Gangl, one of the co-authors. — This value indicates the regions where a local change of the material will lead to the decrease of the objective function". published
P. S. And remember, only by changing their consumption — together we change the world! ©
Join us in Facebook , Vkontakte, Odnoklassniki
Source: ecotechnica.com.ua/technology/578-effektivnost-elektromotora-uvelichena-za-schet-novogo-metoda-optimizatsii-formy.html
To achieve this goal, automotive industry and aircraft industry often rely on filtering – a method that uses simulation to create the most simple and effective used in production designs. "Smoother rotation of the rotor can increase the energy efficiency of the engine and, at the same time, to reduce undesirable side effects, such as noise and vibration," says the mathematician Ulrich Langer (Ulrich Langer).
Professor Kepler University Linz Ulrich Langer, in collaboration with scientists, colleagues from Germany and Austria are the co-authors of scientific work published in the journal SIAM Journal on Scientific Computing, which describes the possibility of improving the performance of electric motors with optimized forms. "The optimal geometry of the motor, which previously was not possible even to imagine, you can now determine," says Langer.

The optimization problem of the form, as a rule, are solved by minimizing a cost function – a mathematical formula that predicts the loss in accordance with the process. Lehner and his colleagues have used this method for interior permanent magnet (IPM) brushless motor, versions of which are sometimes used in washing machines, cooling fans, computers, etc., the Rotor includes iron core and permanent magnets. And since the geometry of all components of the rotor can be changed, the authors highlighted a subregion in the iron core of the rotor, the form of which may be subject to optimization. The result of the design, which allows to get more smooth and efficient rotation.
Scientists proposed an optimization procedure derived from the methods of Lagrange for the approximation of nonlinear problems, and demonstrate effective and accurate means of calculating the shape derivative from the cost function. This simple and comprehensive method allows to solve nonlinear differential equation with partial derivatives (PDE) and total cost functions. Ultimately, their optimization procedure is able to show a 27 percent decrease on the cost function IPM brushless motors, as demonstrated in a specific example of scientific work.
Now, when they effectively used the derivative of the shape optimization, the researchers hope to improve their method with further experiments. "Currently, we are working to create so-called "topological derivative" to solve such nonlinear optimization problems, says Peter Gangl, one of the co-authors. — This value indicates the regions where a local change of the material will lead to the decrease of the objective function". published
P. S. And remember, only by changing their consumption — together we change the world! ©
Join us in Facebook , Vkontakte, Odnoklassniki
Source: ecotechnica.com.ua/technology/578-effektivnost-elektromotora-uvelichena-za-schet-novogo-metoda-optimizatsii-formy.html
Why publicize your relationship on social networks is a mistake?
Recipe burning cocktail for cellulite