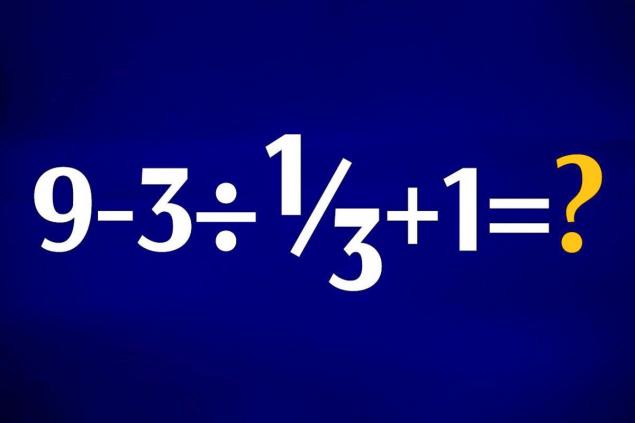An elementary example around which citizens do not get tired of arguing

Teachers struggled for a long time to form reliable mathematical knowledge. Unfortunately, most of them disappeared immediately after the exams. Therefore, simple children's tasks and baffle wise life experience adults. You don't? See if you have the knowledge to handle our examples.



Peels Mathematical Knowledge

1. Who doesn’t like a good online conversation? It doesn’t matter, the battle is between supporters of kvass and kefir or an attempt to determine the color of a mysterious dress. There would be a reason to speak out and smash the arguments of the opposing side to dust.
   
   
   
   And this example also provides such an opportunity. On Facebook, 16,000 people have already commented. For the most impatient of them, the answer was ready in a second: of course, 1! But, as they say, hurry up and make people laugh.
   
   
2. This simple example became famous in Japan and then gained popularity around the world thanks to social media. The authors point out that in the 80s, 90% of students could solve it without problems. Today, less than half of them.
   
   
   
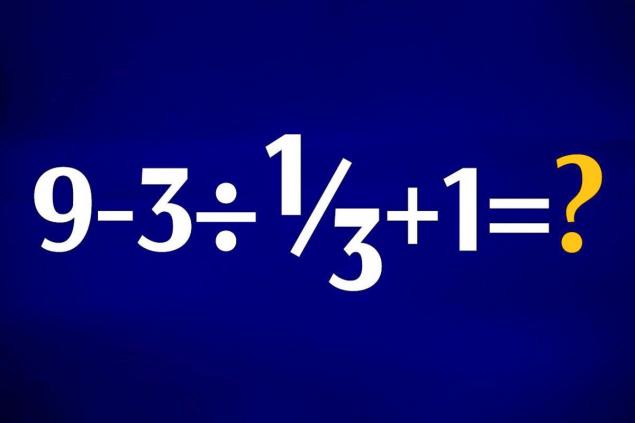
3. Some will say that this example is too simple. But I can assure you that the astonishing abundance of answers in the comments clearly suggests otherwise.
   
   
   

Answers.

1. As you can see, you will have to dust off the forgotten order of mathematical actions. We all taught him in elementary school. And today, many people know thanks to the mnemonic rule of PEMDAS: brackets, degree, multiplication, division, addition, subtraction.
   
   
   
   “That’s what we thought,” the authors of “1” challenged. And they explain: "First action in brackets, then multiplication and lastly division." The devil is known to be in the details. And this example is no exception.
   
   Division and multiplication have the same priority. So you have to do it. left. From this we get 6: 2 (1 + 2) = 6: 2 * 3 = 3 * 3 = 9..
   
   
2. The main problem here is that many simply do not remember how to divide a number by fraction. But this mathematical operation will be the first. First you need to divide and multiply, and only then add and subtract.
   
   
   
   Dividing a number by a fraction is like multiplying it by the same fraction, having previously changed the numerator and denominator places. Then our example gets a more understandable form: 9 - 3 * 3 + 1 = 9 - 9 + 1 = 1st.
   
   

As we have already said, arithmetic actions are performed in turn from left to right. At the same time, first of all, actions are done in brackets, then division and multiplication, and only then addition and subtraction. In words, simple, but in practice, problems arise. Where would readers get answers 0 and 3?



According to the rules, first we perform actions in brackets. 5 - 4 + 1 = 2. Then 18/3 * (5 - 4 + 1) = 18/3 * 2. And here lies another insidious underwater rock. In theory, division can be denoted by a colon (:), an obelus (÷), a slash (/) or a fractional sign (-).

With computer input, the fraction sign is often replaced with a slash for simplicity. Therefore, many people mistakenly perceive the expression “18 / 3 * 2” as a fraction, where in the numerator 18, and in the denominator 3 * 2. And this would be true if our example had the form 18 / (3 * (5 - 4 + 1)). But there is no parenthesis behind the division sign, which means that it cannot be perceived as a fractional trait. Hence 18 / 3 * 2 = 6 * 2 = 12..



I hope you enjoyed taking examples like this. Don’t forget to write down the answers you got. Did we really make a mistake somewhere?