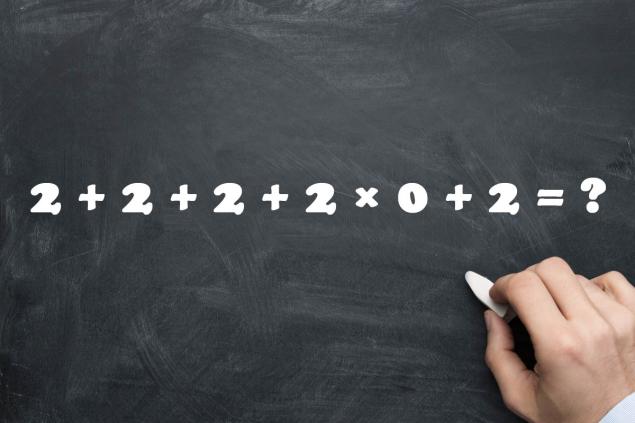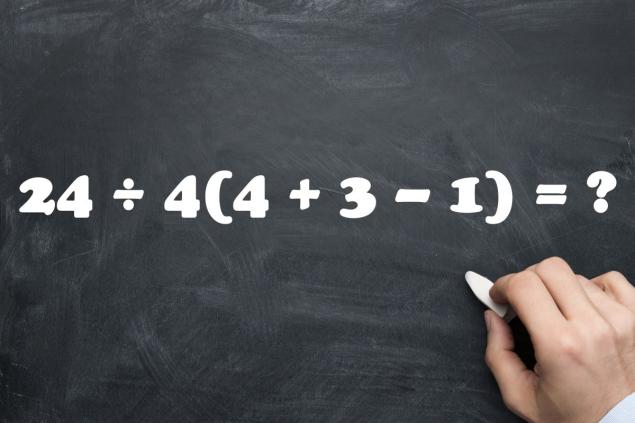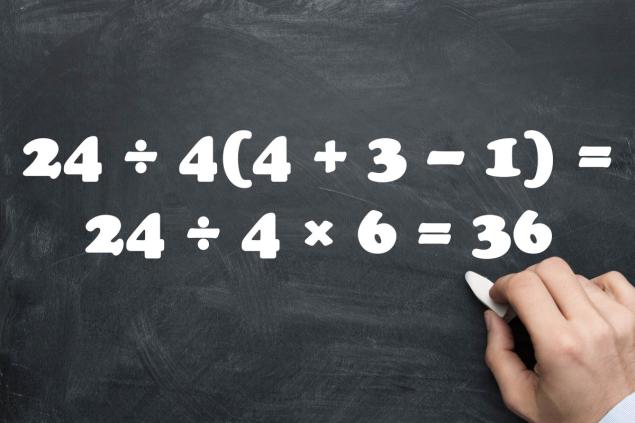Stephen Hawking will make you move the convolutions, but decide without the calculator Bashny.Net

Taking examples of action What every student spends more than a dozen hours on. However, after successfully passed tests and exams, all acquired knowledge is weathered with frightening rapidity. And it would seem that so much work has been invested in the study, but soon there is no trace of all these wisdoms.

Today's edition. "Site" It offers to check how well you mastered the basic algorithms for solving examples. Among other things, there will even be an example attributed to the legendary English scientist Stephen Hawking.



Taking examples of action

1. The first example is likely to be taken by many. If you know all the necessary rules, then you will definitely not get confused in this abundance of twos. Although multiplication by zero can confuse all cards. Be careful with every arithmetic operation.
   
   
   
   
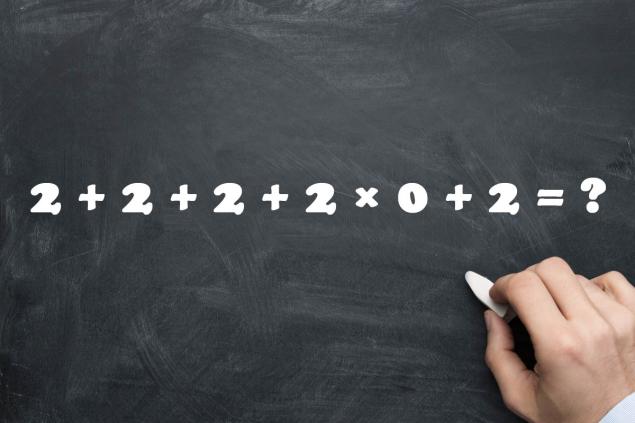
2. In the second mathematical example, in addition to addition and multiplication, you also have to divide. It's not going to be a big problem for you. Still, try to solve without mistakes, remembering along the way those rules that were often repeated by a strict mathematician.
   
   
   
   
3. The latter example (and its various variations) is credited to Stephen Hawking himself. And there really is something brilliant about it. After all, how else to explain that there is always a heated debate around this example. And every reader is sure that his decision of the example is correct. The correct answer, of course, is only one.
   
   
   
   

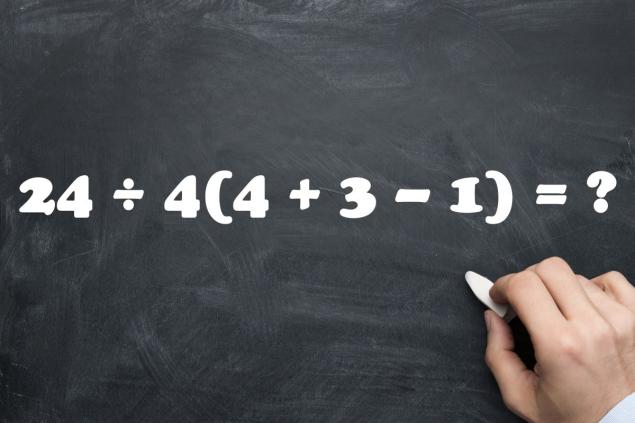
Tips and solutions

1. Many people get a deuce here (both as an example and as an estimate), since all arithmetic operations are performed strictly sequentially from left to right. But real high school students know that multiplication is done here first, not addition. So 2 + 2 + 2 + 2 × 0 + 2 = 2 + 2 + 2 + 0 + 2 = 8.
   
   
   
   
2. The golden rule “first perform division and multiplication, and then addition and subtraction” is useful in solving the second example. Then 5 + 5 ÷ 5 + 3 × 3 = 5 + 1 + 9 = 15.
   
   
   
   
3. Here, first of all, you will have to perform actions in brackets. And then, when the brackets are open, go strictly from left to right. And in this case we get: 24 ÷ 4(4 + 3 - 1) = 24 ÷ 4 × 6 = 36. Many people get one, but this answer is wrong.
   
   
   
   

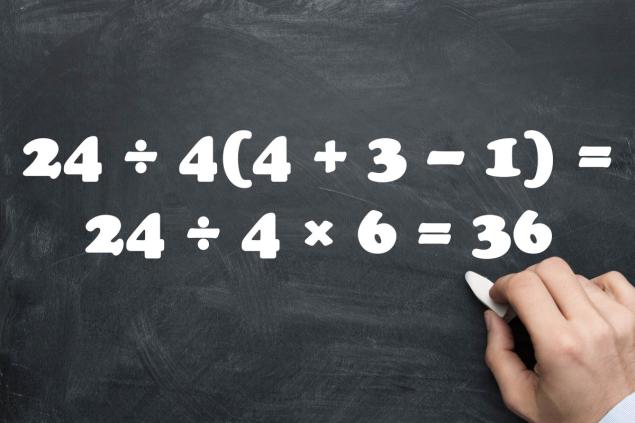
Don’t forget to write down the answers you got and whether they matched ours. Also solve our more complex examples. Or look for solutions to the curious puzzles that we published recently. Such a warm-up for the mind will definitely be useful.