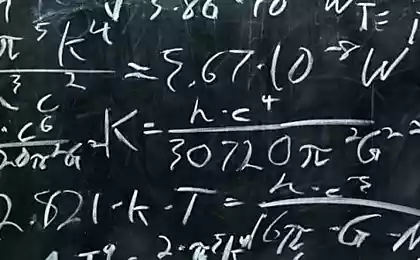Japanese mathematician was able to prove the ABC conjecture

In August this year, the network has the work of the Japanese mathematician named Shinichi Mochizuki. According to Mochizuki, he was able to prove the ABC-conjecture, but only now came the first comments on this work, according to Nature News.

Recall that this hypothesis is considered to be one of the key statements in the theory of numbers. As you know, for the formulation of ABC-hypothesis must be the concept of radical (rad natural number N - a number that is the product of distinct prime divisors of N).

For example, rad (18) is equal to 6, t. To. At number 18, two prime divisors - 3 and 2, the product is equal to 6.

The work of scientists took more than 500 pages, ie. A. To prove the ABC-conjecture he decided to build a new theory - the arithmetic version of the theory of Teichmüller spaces.

According to experts, currently in the proof did not reveal any major gaps, and held Mochizuki work can be instrumental in the study of a variety of other complex mathematical problems.

In his work Mochizuki partially used object that is used in the proof of Andrew Wiles Fermat's Last Theorem, and if the evidence Mochizuki be true, then it will immediately follow and Fermat's Last Theorem.