686
Bee savings: why does nature prefer hexagons?
With enough observation in nature easily detect the strict geometry. A special honor to be hexagons — regular hexagons.
Why are they so fond of bees and architects, and what are their advantages from the point of view of physics, said a British scientist and science journalist Philip ball.
Here is the excerpt from the book "Patterns in nature: Why the living world looks like", published on the website of Nautilus.

As the bees do it? Honeycombs in which they store Golden nectar, are miracles of engineering, recruitment of cells in the form of a prism with the correct hexagon in the base. The thickness of the wax walls is strictly defined, cells slightly deviate from the horizontal, so that the viscous honey does not flow and the cell are in balance with the influence of Earth's magnetic field. But this design without drawings and forecasts build a lot of bees who are, and somehow coordinate their attempts to make a honeycomb the same.
The ancient Greek philosopher Papp Alexandria thought that bees must be endowed with "geometric insight." And who if not the Lord would give them this wisdom? As wrote the English entomologist William Kirby in the mid-nineteenth century, bees — "mathematics of God." Charles Darwin was not sure about this and conducted experiments to determine if bees can build a perfect honeycomb using only the acquired and innate abilities, as was assumed in his theory of evolution.
But still, why a hexagon? This is a purely geometric question. If you want to put together several identical in shape and dimensions so that it fills the entire plane, suitable only three correct figure (with equal sides and angles):
If you choose these options, the hexagonal honeycomb will require the smallest total length of partitions, unlike the triangles and squares of the same area. Therefore, in the bee of love to hexagonal makes sense: for the manufacture of wax is wasted energy, and they try to minimize costs — just as the builders trying to save on the cost of bricks. To such conclusion came in the eighteenth century, and Darwin announced that honeycomb of regular hexagons "is ideal for economy of labor and wax".
Darwin thought that natural selection gave the bees instincts for the construction of wax cells, which have a significant advantage: they need to spend less time and energy than in other cell forms. And although it seems that bees do possess special abilities in terms of measuring angles and thickness of walls, opinions among scientists about the extent to which insects they are used, varies because of the clusters of hexagons occur in nature quite often.
If you blow on the bubbles on the surface of the water to corral them together, they will take the form of hexagons — or at least closer to it.
You will never see the Assembly square bubbles: even if the four walls are in contact, they immediately rebuilt in the design with three sides, between which are about equal angles of 120 degrees — something like a center emblem of a Mercedes.
Obviously, there are no organisms that would work on these glued bubbles, like bees over honeycombs. The figure is formed exclusively by the laws of physics. It is also clear that these laws have certain preferences: for example, the tendency to a triangular connection of the walls of the bubbles. The same thing happens with the foam, which is complicated in structure.
If you blow through a straw into soapy water and create a "mountain" of bubbles in three-dimensional space, you can see that their walls are in contact always provide the quadripartite Union and intersecting of the membrane are at an angle of about 109 degrees is the angle that is directly related to the tetrahedron.
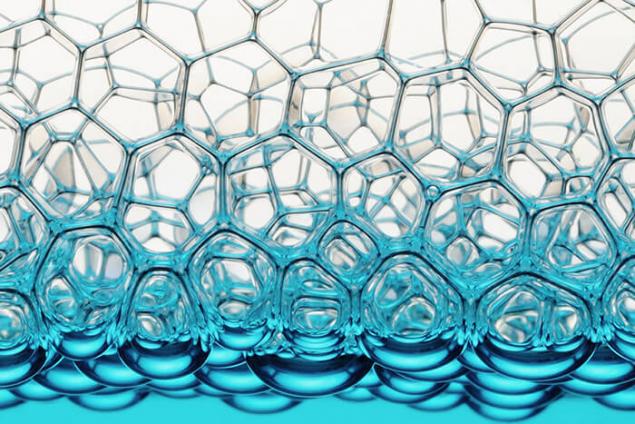
What determines the form of bubbles and formation of "forks" soap walls? Nature is still more concerned with saving than bees. Bubbles and soap film consists of water (and layers of soap molecules) and surface tension compresses the surface of the liquid so that it occupied the smallest area. Therefore, drops of rain in the fall take the form close to spherical: the sphere has the smallest surface area compared to other shapes of the same volume. On wax leaf water drops are compressed into small beads for the same reason.
Surface tension explains the pattern formed by bubbles or foam. Foam is committed to this design, in which the total surface tension will be minimal, and therefore, the minimum should be and the area of the soap membrane. But the configuration of the walls of the bubbles should be durable from the point of view of mechanics: tension in different directions at the "crossroads" should be perfectly balanced (on the same principle the right balance in the construction of the walls of the Cathedral). Three way connection in the film of the bubbles, and four-sided — foam — combinations that achieve this balance.
But those who think (and there are such) that honeycomb is simply the abundance of frozen bubbles of warm wax, it will be difficult to explain how the same plurality of hexagonal cells are obtained from paper wasps, which are used in the construction of not wax and lumps of chewed-up wood fibers and stems, from which they made a kind of paper. Moreover, the surface tension here does not play a role, but also clear that different types of OS, different innate instincts, in terms of architectural decisions: they can vary considerably.
Although the geometry of the joints of the walls of the bubbles is dictated by the interaction of mechanical forces, it is pointless to look for a hint at what form should accept foam. Regular foam contains a multi-faceted elements of varying shape and size. Look closely and you will see that their walls are not perfectly straight: they are slightly curved. Because the smaller the bubble, the higher the gas pressure, the wall of the small bubble next to the large will slightly bulge forward. Moreover, some elements of the five faces, others six, and some only four or only three. With a little flexibility of the walls of all of these shapes can form four-sided compound, similar in composition to the tetrahedron, which is necessary for mechanical stability. So the shape of the bubbles may vary. Although the foam can be studied using the rules of geometry, at its core, it is pretty chaotic.
Suppose you could make the "perfect" foam in which all bubbles are the same size. What should be their ideal form, to the total area of the walls was the lowest, but the demand for corners at the intersection are met? This issue has been discussed for many years, and has long been considered that the ideal form is chetyrnadcatiletnii c square and hexagonal faces. However, in 1993 was opened a little more economical, though less ordered structure, consisting of repeating groups of eight different forms.
This more complex pattern was used as inspiration for Prepodobnogo design water stadium for the Olympics 2008 in Beijing.

The building of the National swimming center in Beijing
Rules running for bubbles in the foam can also be attributed to other patterns that are found in living organisms. Not only compound eyes of flies are composed of groups of hexagonal cells that resemble groups of bubbles; still light-sensitive cells in each of these cells are collected in clusters of four, which again is reminiscent of soap bubbles. Even in the case of flies-mutants, in which such cells are more possible to speak about what their organization is more or less identical to the behavior of the bubbles.
Due to the surface tension of a soap film spanning a wire loop is stretched straight as an elastic mesh of the trampoline. But if the wire frame is bent, the film will also dish out the elegant contour, which automatically tells you the most economical from the point of view of material utilization method of the coating space, enclosed with a frame. Thus, the architect can see how to build a roof for a building with complex architecture and to spend a minimum of materials. Anyway, it's not just the economy these so-called minimal surfaces, but in their beauty and elegance; which is why architects like Frei Otto, used them as inspiration for their works.
These surfaces minimize not only area but also curvature. The steeper the curve, the greater the curvature. It can be positive (protuberances) or negative (cavity or trough). The average curvature of a curved surface will be zero if positive and negative curvature balance each other. Therefore, the sheet can be covered with curvature and the average curvature will be lower. Such a minimally curved surface cuts space neat maze of corridors and channels network.

Frei Otto, Olympic stadium in Munich © Atelier Frei Otto Warmbronn
This phenomenon is called periodic minimal surface ("periodic" simply means that this structure is repeated again and again; in other words, it is a constant sequence). When such sequences were discovered in the nineteenth century, they seemed to be just a mathematical curiosity. But now we know that nature can benefit from.
Cells of organisms of different species, from plants to lampreys or rats, have membranes with similar microscopic structures. No one knows why they are needed, but they are so common that it is logical to assume that they perform some useful function. Maybe they separate one biochemical process from another, abolishing their mutual influence on each other. Or perhaps they are simply effective as a "working surface", as many biochemical processes occur in the membranes where enzymes and other active molecules. Whatever the function of these labyrinths, you do not need a complex genetic instructions for their construction: the laws of physics will do everything for you.
Some butterflies such as phengaris Mattila, on the wings have scales, which is a neat maze of tough material — chitin, is formed in the form of certain periodic minimal surface called gyroid. The interaction between irregularities on the scaly surface of the wings leads to the fact that waves of a certain length — that is, certain colors disappear, while others reinforce each other. This mechanism affects the color of the insect.
The skeleton of the sea urchin Cidaris rugosa — porous set of cells in the form of another periodic minimal surface. It is an exoskeleton that is outside the soft tissues of the body, protective shell, which grow seemingly dangerous spines from the same mineral which enters into the composition of chalk and marble. The open lattice structure indicates that the material is durable, but not heavy, as the foamed metal used in aircraft building.

To create an ordered structure from solid stubborn mineral, these organisms apparently do a mock-up of a soft bendable membrane and then crystallized solid substance inside one of the interpenetrating networks.
Other creatures can use the mineral foam for more complex tasks. From it they build design-"the trellis", which, as the mirrors can direct the light due to its reflection from the terrain. Network of hollow microscopic channels, honeycomb, chitinous bristles extraordinary sea worm (sea mouse) turns these volosataya structure in natural optical fiber, which may refract light, making the color of the substance can change from red to bluish-green depending on the lighting direction. The color change helps to scare off predators.
This principle is the use of soft tissues and membranes as the layout for the formation of an ordered mineral exoskeleton is widely distributed among marine life. Some sea sponges have an exoskeleton made of mineral cores connected on the principle of "webs" on the playgrounds, and they are incredibly reminiscent of the shapes, which are formed in the collision of soap bubbles in foam, and there can be no talk about coincidences, because the architecture dictates the surface tension.
A similar process known as biomineralisation, produce a spectacular result in such marine organisms as a pod and diatoms. Some of them are lined up neatly exoskeletons, consisting of mineral cells in the form of hexagons and pentagons: they can be called sea combs.
Also interesting: the geometry of the about life — JUST about the COMPLEX!
Fractal geometry and genetic code of the Universe
When the German scientist (and talented artist) Ernst Haeckel first saw these forms in the microscope in the late nineteenth century, he made them the chief ornament of his collection of drawings called "the Beauty of forms in nature", which greatly influenced artists of the early XX century and is still admired. For Haeckel, this design was a proof of the fundamental creativity of nature — preference for order and patterns embedded in the very laws of nature.
Even if today we do not subscribe to this theory, there is something in this conviction of Haeckel in that order is the irrepressible impulse of the living world, and we can consider it beautiful.published
Author: Donskaya Ksenia
Source: theoryandpractice.ru/posts/15216-pchelinaya-ekonomiya-pochemu-priroda-predpochitaet-shestiugolniki
Why are they so fond of bees and architects, and what are their advantages from the point of view of physics, said a British scientist and science journalist Philip ball.
Here is the excerpt from the book "Patterns in nature: Why the living world looks like", published on the website of Nautilus.

As the bees do it? Honeycombs in which they store Golden nectar, are miracles of engineering, recruitment of cells in the form of a prism with the correct hexagon in the base. The thickness of the wax walls is strictly defined, cells slightly deviate from the horizontal, so that the viscous honey does not flow and the cell are in balance with the influence of Earth's magnetic field. But this design without drawings and forecasts build a lot of bees who are, and somehow coordinate their attempts to make a honeycomb the same.
The ancient Greek philosopher Papp Alexandria thought that bees must be endowed with "geometric insight." And who if not the Lord would give them this wisdom? As wrote the English entomologist William Kirby in the mid-nineteenth century, bees — "mathematics of God." Charles Darwin was not sure about this and conducted experiments to determine if bees can build a perfect honeycomb using only the acquired and innate abilities, as was assumed in his theory of evolution.
But still, why a hexagon? This is a purely geometric question. If you want to put together several identical in shape and dimensions so that it fills the entire plane, suitable only three correct figure (with equal sides and angles):
- equilateral triangles
- squares
- hexagons.
If you choose these options, the hexagonal honeycomb will require the smallest total length of partitions, unlike the triangles and squares of the same area. Therefore, in the bee of love to hexagonal makes sense: for the manufacture of wax is wasted energy, and they try to minimize costs — just as the builders trying to save on the cost of bricks. To such conclusion came in the eighteenth century, and Darwin announced that honeycomb of regular hexagons "is ideal for economy of labor and wax".
Darwin thought that natural selection gave the bees instincts for the construction of wax cells, which have a significant advantage: they need to spend less time and energy than in other cell forms. And although it seems that bees do possess special abilities in terms of measuring angles and thickness of walls, opinions among scientists about the extent to which insects they are used, varies because of the clusters of hexagons occur in nature quite often.
If you blow on the bubbles on the surface of the water to corral them together, they will take the form of hexagons — or at least closer to it.
You will never see the Assembly square bubbles: even if the four walls are in contact, they immediately rebuilt in the design with three sides, between which are about equal angles of 120 degrees — something like a center emblem of a Mercedes.
Obviously, there are no organisms that would work on these glued bubbles, like bees over honeycombs. The figure is formed exclusively by the laws of physics. It is also clear that these laws have certain preferences: for example, the tendency to a triangular connection of the walls of the bubbles. The same thing happens with the foam, which is complicated in structure.
If you blow through a straw into soapy water and create a "mountain" of bubbles in three-dimensional space, you can see that their walls are in contact always provide the quadripartite Union and intersecting of the membrane are at an angle of about 109 degrees is the angle that is directly related to the tetrahedron.

What determines the form of bubbles and formation of "forks" soap walls? Nature is still more concerned with saving than bees. Bubbles and soap film consists of water (and layers of soap molecules) and surface tension compresses the surface of the liquid so that it occupied the smallest area. Therefore, drops of rain in the fall take the form close to spherical: the sphere has the smallest surface area compared to other shapes of the same volume. On wax leaf water drops are compressed into small beads for the same reason.
Surface tension explains the pattern formed by bubbles or foam. Foam is committed to this design, in which the total surface tension will be minimal, and therefore, the minimum should be and the area of the soap membrane. But the configuration of the walls of the bubbles should be durable from the point of view of mechanics: tension in different directions at the "crossroads" should be perfectly balanced (on the same principle the right balance in the construction of the walls of the Cathedral). Three way connection in the film of the bubbles, and four-sided — foam — combinations that achieve this balance.
But those who think (and there are such) that honeycomb is simply the abundance of frozen bubbles of warm wax, it will be difficult to explain how the same plurality of hexagonal cells are obtained from paper wasps, which are used in the construction of not wax and lumps of chewed-up wood fibers and stems, from which they made a kind of paper. Moreover, the surface tension here does not play a role, but also clear that different types of OS, different innate instincts, in terms of architectural decisions: they can vary considerably.
Although the geometry of the joints of the walls of the bubbles is dictated by the interaction of mechanical forces, it is pointless to look for a hint at what form should accept foam. Regular foam contains a multi-faceted elements of varying shape and size. Look closely and you will see that their walls are not perfectly straight: they are slightly curved. Because the smaller the bubble, the higher the gas pressure, the wall of the small bubble next to the large will slightly bulge forward. Moreover, some elements of the five faces, others six, and some only four or only three. With a little flexibility of the walls of all of these shapes can form four-sided compound, similar in composition to the tetrahedron, which is necessary for mechanical stability. So the shape of the bubbles may vary. Although the foam can be studied using the rules of geometry, at its core, it is pretty chaotic.
Suppose you could make the "perfect" foam in which all bubbles are the same size. What should be their ideal form, to the total area of the walls was the lowest, but the demand for corners at the intersection are met? This issue has been discussed for many years, and has long been considered that the ideal form is chetyrnadcatiletnii c square and hexagonal faces. However, in 1993 was opened a little more economical, though less ordered structure, consisting of repeating groups of eight different forms.
This more complex pattern was used as inspiration for Prepodobnogo design water stadium for the Olympics 2008 in Beijing.

The building of the National swimming center in Beijing
Rules running for bubbles in the foam can also be attributed to other patterns that are found in living organisms. Not only compound eyes of flies are composed of groups of hexagonal cells that resemble groups of bubbles; still light-sensitive cells in each of these cells are collected in clusters of four, which again is reminiscent of soap bubbles. Even in the case of flies-mutants, in which such cells are more possible to speak about what their organization is more or less identical to the behavior of the bubbles.
Due to the surface tension of a soap film spanning a wire loop is stretched straight as an elastic mesh of the trampoline. But if the wire frame is bent, the film will also dish out the elegant contour, which automatically tells you the most economical from the point of view of material utilization method of the coating space, enclosed with a frame. Thus, the architect can see how to build a roof for a building with complex architecture and to spend a minimum of materials. Anyway, it's not just the economy these so-called minimal surfaces, but in their beauty and elegance; which is why architects like Frei Otto, used them as inspiration for their works.
These surfaces minimize not only area but also curvature. The steeper the curve, the greater the curvature. It can be positive (protuberances) or negative (cavity or trough). The average curvature of a curved surface will be zero if positive and negative curvature balance each other. Therefore, the sheet can be covered with curvature and the average curvature will be lower. Such a minimally curved surface cuts space neat maze of corridors and channels network.

Frei Otto, Olympic stadium in Munich © Atelier Frei Otto Warmbronn
This phenomenon is called periodic minimal surface ("periodic" simply means that this structure is repeated again and again; in other words, it is a constant sequence). When such sequences were discovered in the nineteenth century, they seemed to be just a mathematical curiosity. But now we know that nature can benefit from.
Cells of organisms of different species, from plants to lampreys or rats, have membranes with similar microscopic structures. No one knows why they are needed, but they are so common that it is logical to assume that they perform some useful function. Maybe they separate one biochemical process from another, abolishing their mutual influence on each other. Or perhaps they are simply effective as a "working surface", as many biochemical processes occur in the membranes where enzymes and other active molecules. Whatever the function of these labyrinths, you do not need a complex genetic instructions for their construction: the laws of physics will do everything for you.
Some butterflies such as phengaris Mattila, on the wings have scales, which is a neat maze of tough material — chitin, is formed in the form of certain periodic minimal surface called gyroid. The interaction between irregularities on the scaly surface of the wings leads to the fact that waves of a certain length — that is, certain colors disappear, while others reinforce each other. This mechanism affects the color of the insect.
The skeleton of the sea urchin Cidaris rugosa — porous set of cells in the form of another periodic minimal surface. It is an exoskeleton that is outside the soft tissues of the body, protective shell, which grow seemingly dangerous spines from the same mineral which enters into the composition of chalk and marble. The open lattice structure indicates that the material is durable, but not heavy, as the foamed metal used in aircraft building.

To create an ordered structure from solid stubborn mineral, these organisms apparently do a mock-up of a soft bendable membrane and then crystallized solid substance inside one of the interpenetrating networks.
Other creatures can use the mineral foam for more complex tasks. From it they build design-"the trellis", which, as the mirrors can direct the light due to its reflection from the terrain. Network of hollow microscopic channels, honeycomb, chitinous bristles extraordinary sea worm (sea mouse) turns these volosataya structure in natural optical fiber, which may refract light, making the color of the substance can change from red to bluish-green depending on the lighting direction. The color change helps to scare off predators.
This principle is the use of soft tissues and membranes as the layout for the formation of an ordered mineral exoskeleton is widely distributed among marine life. Some sea sponges have an exoskeleton made of mineral cores connected on the principle of "webs" on the playgrounds, and they are incredibly reminiscent of the shapes, which are formed in the collision of soap bubbles in foam, and there can be no talk about coincidences, because the architecture dictates the surface tension.
A similar process known as biomineralisation, produce a spectacular result in such marine organisms as a pod and diatoms. Some of them are lined up neatly exoskeletons, consisting of mineral cells in the form of hexagons and pentagons: they can be called sea combs.
Also interesting: the geometry of the about life — JUST about the COMPLEX!
Fractal geometry and genetic code of the Universe
When the German scientist (and talented artist) Ernst Haeckel first saw these forms in the microscope in the late nineteenth century, he made them the chief ornament of his collection of drawings called "the Beauty of forms in nature", which greatly influenced artists of the early XX century and is still admired. For Haeckel, this design was a proof of the fundamental creativity of nature — preference for order and patterns embedded in the very laws of nature.
Even if today we do not subscribe to this theory, there is something in this conviction of Haeckel in that order is the irrepressible impulse of the living world, and we can consider it beautiful.published
Author: Donskaya Ksenia
Source: theoryandpractice.ru/posts/15216-pchelinaya-ekonomiya-pochemu-priroda-predpochitaet-shestiugolniki























