166
Soviet schoolchildren of the 50s coped with this problem in a couple of minutes, check how long it will take you
Development of Mathematical Thinking It is impossible without solving difficult problems. But how do you know which task is difficult? After all, the same task is impossible for some and too easy for others. Therefore, our puzzles and other tasks are almost always taken from the school curriculum. After all, if students decide this, then our adult readers will be able to find a solution.

And today's edition. "Site" It will offer an old problem of Soviet times. I wonder how many people will cope and find a sum of fractions here. Will it be possible to at least guess what kind of path you need to go to get to the right decision faster?
Of course, many will remember that to find the sum of fractions, you need to come to a common denominator. But in today's assignment, it's quite difficult. Just spend a lot of time and effort. And you can always make a mistake that will nullify all efforts. Still, look at this assignment and think about what you can do with it.
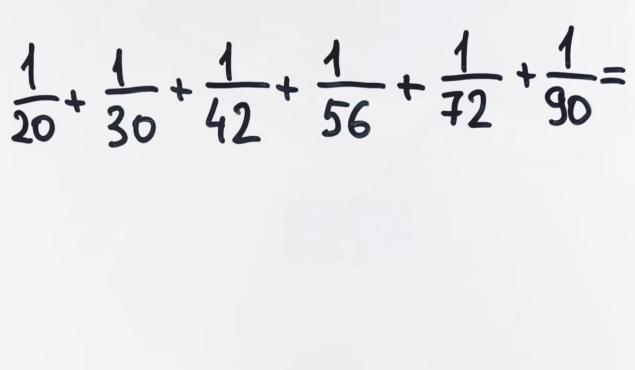
An experienced mathematician shows his method of solution. To do this, he first arranges all denominators into multipliers. After all, the existing numbers are quite suitable for this.
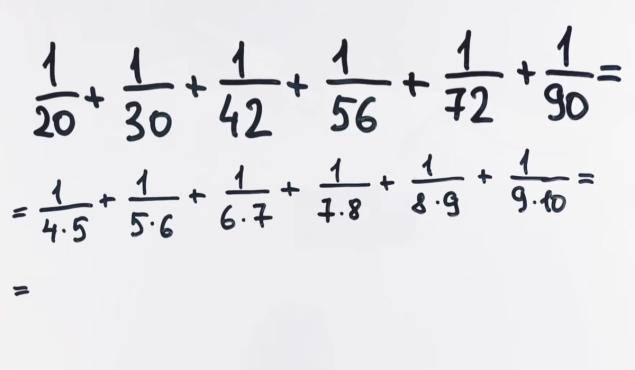
And since we have a unit everywhere in the numerator, we can write it in each case with the same numbers that we have in the denominator. For this in the first case will be 5 – 4, then 6 – 5 and so on.
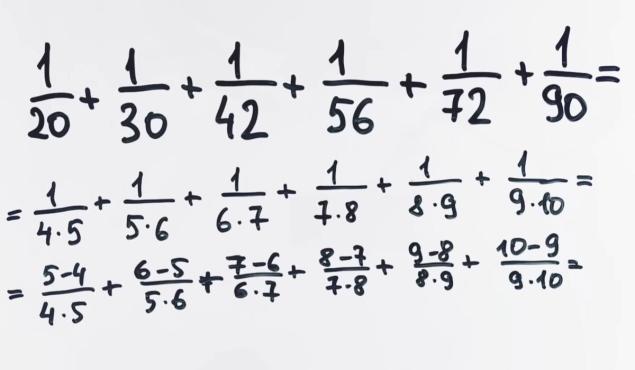
As you can see, the original example has changed dramatically. However, this is only a change of appearance for a simpler solution. The values remain the same, so you can continue with a calm conscience.
Then the author of the decision proposes to divide the numerator by denominator. This will allow us to get closer to the solution, because in every fraction we get, we can cut something.
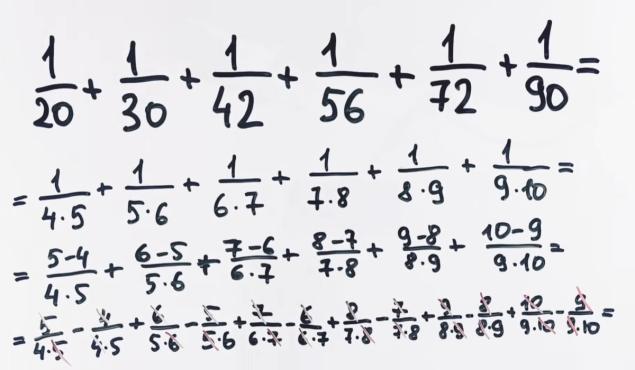
After such clever mathematical manipulations, we will get an almost ready answer. There's not much work left to do. After all, the fractions available after the next sign of equality are reduced and give the answer that we have been looking for all this time. Which initially seemed to be very difficult to find.

Now bring everything to a common denominator to get the final fraction. It was a beautiful decision. The solution to this problem can be seen in more detail on the wonderful channel "This is not taught in school."

Of course, the numbers in this task were originally selected in such a way that it became possible to solve everything in a non-standard way. In this case, there are other ways to come to a decision. Did you make a decision? Tell me exactly how.

And today's edition. "Site" It will offer an old problem of Soviet times. I wonder how many people will cope and find a sum of fractions here. Will it be possible to at least guess what kind of path you need to go to get to the right decision faster?
Of course, many will remember that to find the sum of fractions, you need to come to a common denominator. But in today's assignment, it's quite difficult. Just spend a lot of time and effort. And you can always make a mistake that will nullify all efforts. Still, look at this assignment and think about what you can do with it.
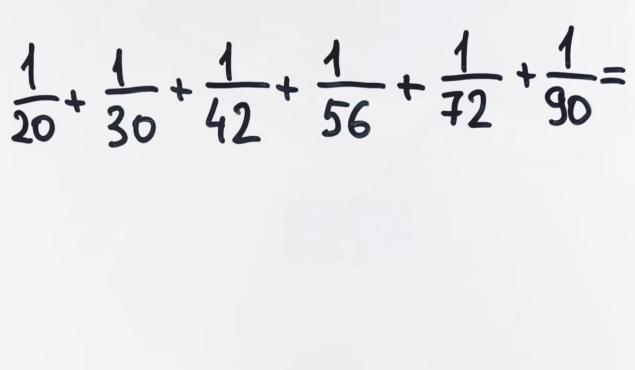
An experienced mathematician shows his method of solution. To do this, he first arranges all denominators into multipliers. After all, the existing numbers are quite suitable for this.
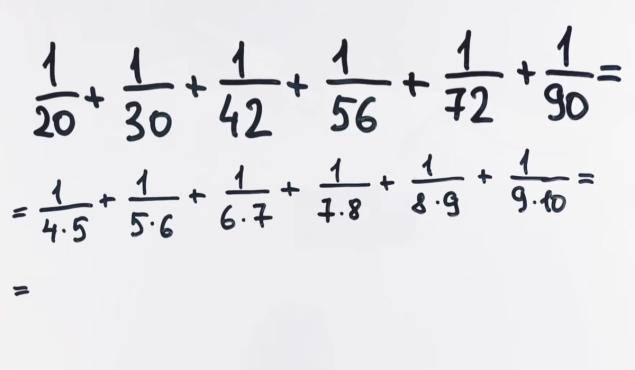
And since we have a unit everywhere in the numerator, we can write it in each case with the same numbers that we have in the denominator. For this in the first case will be 5 – 4, then 6 – 5 and so on.
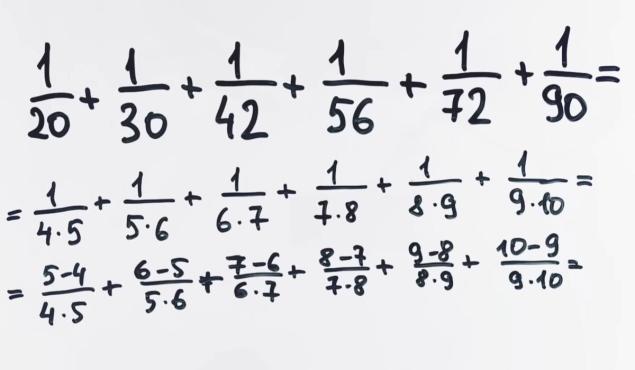
As you can see, the original example has changed dramatically. However, this is only a change of appearance for a simpler solution. The values remain the same, so you can continue with a calm conscience.
Then the author of the decision proposes to divide the numerator by denominator. This will allow us to get closer to the solution, because in every fraction we get, we can cut something.
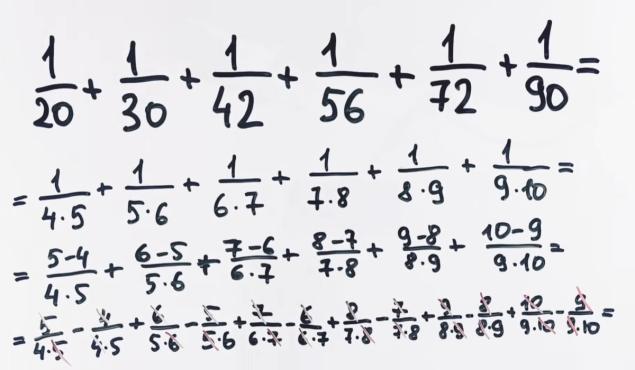
After such clever mathematical manipulations, we will get an almost ready answer. There's not much work left to do. After all, the fractions available after the next sign of equality are reduced and give the answer that we have been looking for all this time. Which initially seemed to be very difficult to find.

Now bring everything to a common denominator to get the final fraction. It was a beautiful decision. The solution to this problem can be seen in more detail on the wonderful channel "This is not taught in school."

Of course, the numbers in this task were originally selected in such a way that it became possible to solve everything in a non-standard way. In this case, there are other ways to come to a decision. Did you make a decision? Tell me exactly how.
Sensors that detect radiation have already arrived, the witch Maria Tikhaya told us what we are preparing for
Psychologists have named three alarming signs by which you can determine the secret Plyushkin, I think I need to talk to my mother-in-law.